Teaching Pythagoras' theorem31/3/2015 I absolutely love teaching Pythagoras' theorem - not sure why, but I suspect it has something to do with the sense of "wow" I got when I first learned about it and realised that maths isn't all about doing sums. There's loads of really interesting stuff you can do with it, and as the first real theorem that most pupils will meet, I think it's worth doing it justice.
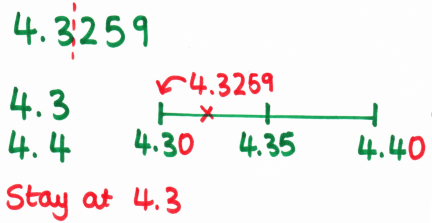
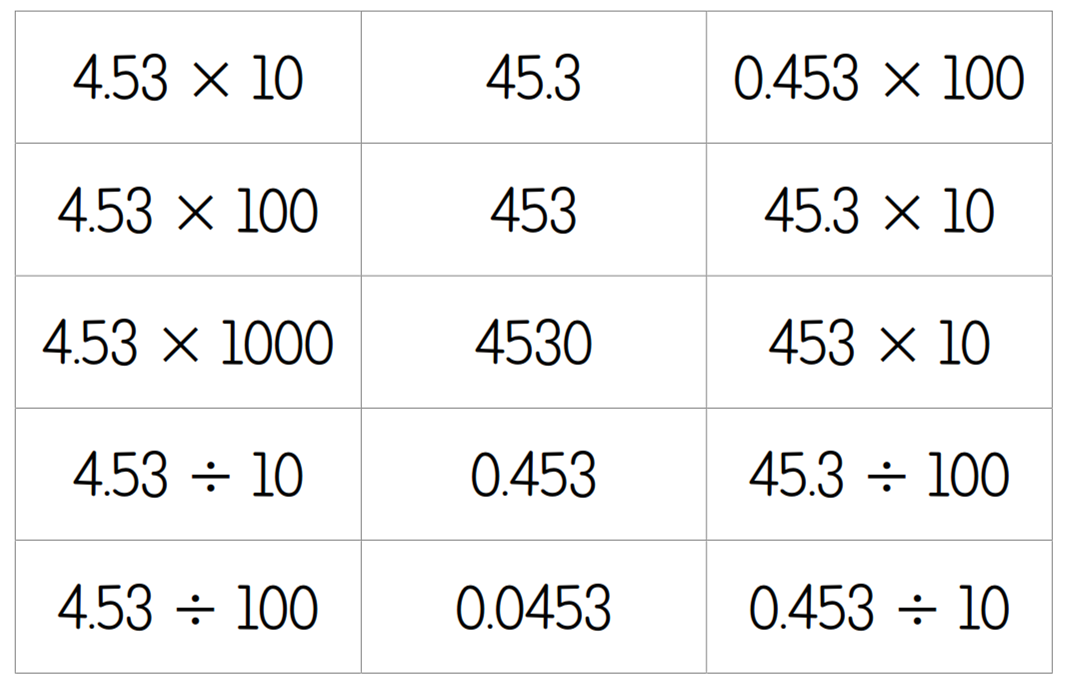
Teaching rounding24/3/2015
Following my trawl through the xkcd archives to find my favourite comics for A Level, I thought I may as well post some that may be relevant for KS3 and GCSE. There are plenty of funny graphs and pie charts (great for the last five minutes of a lesson), and a few topic-related strips.
This post contains a few percentages comics; while they're quite funny, they also have quite a bit of mileage in terms of mathematical discussion, particularly "Hand Sanitizer" and "Fastest Growing". Clicking on each picture will take you to the xkcd site, where you can get larger resolution versions. xkcd strips for A Level22/3/2015 If you haven't heard of xkcd (click now and lose three hours), it's a webcomic run by Randall Monroe focusing on maths, science and computing. Although some of his comics (mostly the coding ones) go over my head, the maths-based ones are hilarious, and some are great for sharing in lessons - with the caveat that some do contain naughty jokes, so check first. Here's my pick of a few particularly relevant to the A Level syllabus:
Pick of Twitter 21/03/1521/3/2015 Predictably, lots of the blog posts I've read this week have been about the third Maths Conference last Saturday. Having said that, it's great to read other people's perspectives and get an idea of what went on in all the workshops I didn't attend. Here's a few I've enjoyed:
It's no secret that I'm a big fan of bar modelling to get pupils to really think about the calculations they are doing. It's a great way to introduce work with percentages too, and solidifies the link between percentage and fraction calculations. One particular advantage is the flexibility it affords - I had one pupil decide that the best way for her to find 15% was to work out 25% and 10%, then subtract one from the other, rather than the more "traditional" method we'd probably all teach of finding 10% and 5%, then adding together.
Mix Match19/3/2015 A great quick activity to get pupils out of their seats; this works particularly well at the end of a lesson for an exit activity and can be used for most topics.
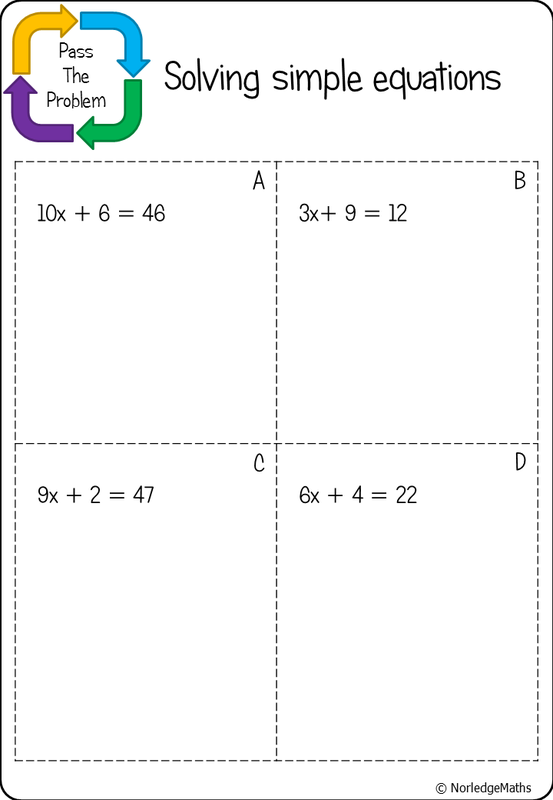
Pass the Problem18/3/2015 This is a great way to get pupils collaborating in the classroom and focusing on the steps to solve problems.
Teaching solving equations17/3/2015 The teaching methods I use for solving equations have changed drastically over my (relatively short) career so far, so here's a post about my journey so far and current "tried and tested" ideas. 1. Why animal algebra doesn't work
One very clear memory I have of my time at school is the first term of work I did in Year 7. My teacher was properly "old-school" - he'd demonstrate for 15 minutes, then we'd work in silence for the remaining 45 minutes on similar problems. He was the deputy head, and we were all pretty terrified of him; while I don't want to debate if that's a good teaching model or not, one thing that really stuck with me was that first term's work - we did nothing but solving equations, pretty much from September until Christmas. It wasn't just simple linear though; we went all the way to solving quadratics using the formula, although I confess I didn't really know what I was doing or why I was doing it. As a result, I've always loved algebra and been pretty good at it (conversely, my geometrical reasoning skills are pretty dire). By the time I teach linear equations, I've already used algebra tiles quite a lot with pupils to create algebraic expressions. After working through one-step equations and ensuring pupils understand the processes involved (rather than just solving "by eye"), I move on to linear equations.
Not sure what algebra tiles are? Start here:
What did you do for Pi Day this year?14/3/2015
This was the first lesson I taught using algebra tiles (you can find out about them here) and it's my go-to way to introduce completing the square now. It's worth starting with a little work on factorising first, just so you don't completely blow their minds.
I discovered algebra tiles last year when, in a fit of desperation, I took to the Internet to find a good way of teaching completing the square in a way that might actually get it to stick in the heads of my Year 11s.
Meaningful feedback on past papers11/3/2015
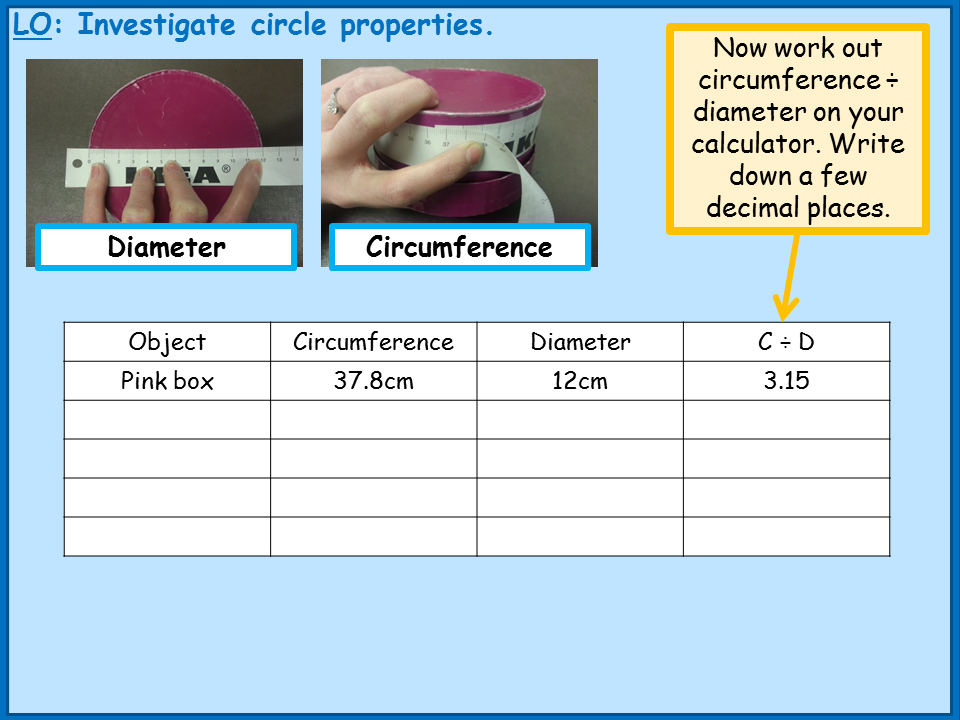
Discovering pi11/3/2015
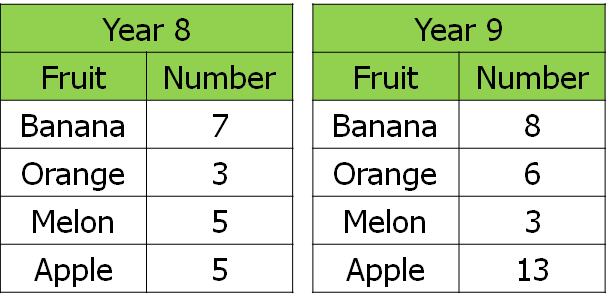
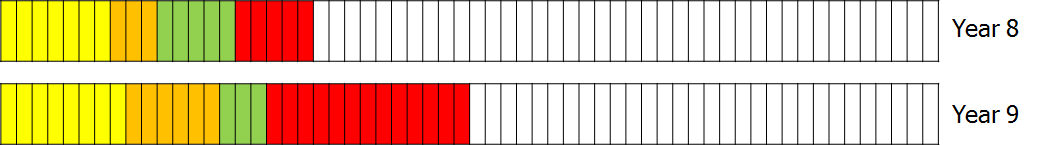
Introducing fraction arithmetic (2)8/3/2015 This is part of a series of blogs on my favourite way to teach adding and subtracting fractions in a way that sticks and really develops understanding of the process. If you haven't already done so, you can read about Lesson 1 here. Lesson 2 - The importance of equal-sized bars At the start of the next lesson, present pupils with two more sets of data, this time comparing two groups of unequal size. Using group sizes of 20 and 30 are particularly effective. I usually get the class to first represent both sets of data on two more bar diagrams using the template from the previous lesson and get them to record the fractions of Year 8s and 9s that preferred each fruit, cancelling down to the simplest form.
Introducing fraction arithmetic (1)8/3/2015
The lesson(s) detailed below have been absolutely groundbreaking for me in terms of teaching adding and subtracting fractions in a way that makes the topic stick and that pupils really understand. The ideas behind it were introduced to me as part of the NCETM's Multiplicative Reasoning course (previously mentioned in my blog about bar modelling). Unfortunately, while I have the lesson materials, I'm still unsure about their status in terms of sharing - they were presented to us as trial materials, with the suggestion that they would be available to schools nationwide once the project had finished, but I can't find them anywhere on the NCETM's website (yet). So although I can't post a link to the PowerPoint and lesson materials I'm using, I thought I'd pop up a quick blog about the ideas behind the materials.
|
Categories
All
Archives
August 2020
|


 RSS Feed
RSS Feed
