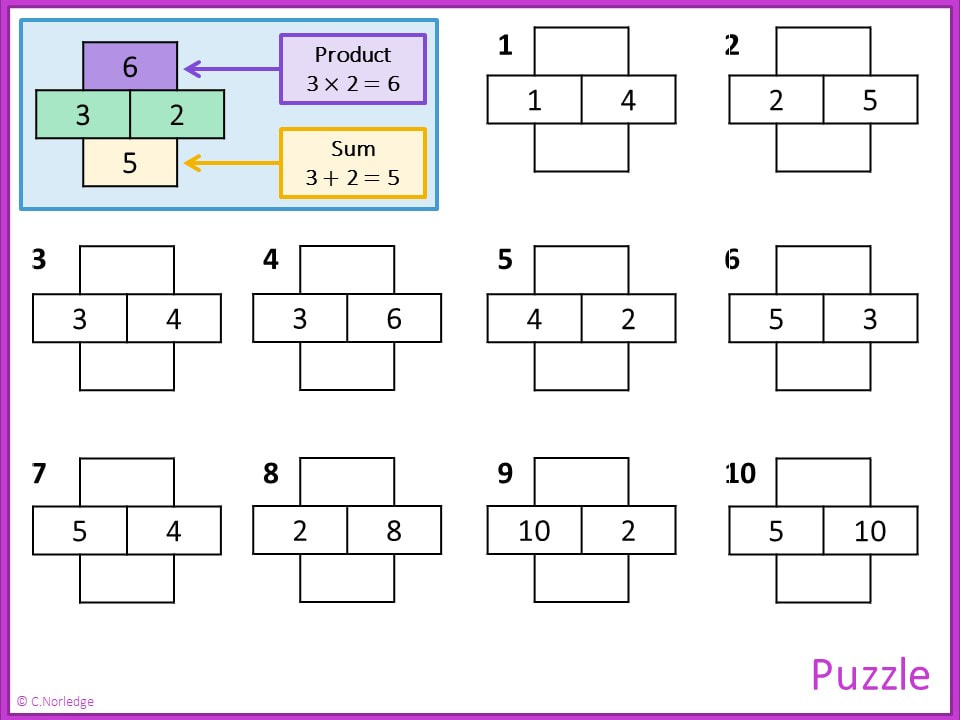
These puzzles are useful as a starter or quick introductory activity for expanding standard quadratic expressions.
|
Working forwards...
These puzzles are useful as a starter or quick introductory activity for expanding standard quadratic expressions.
This was the first lesson I taught using algebra tiles (you can find out about them here) and it's my go-to way to introduce completing the square now. It's worth starting with a little work on factorising first, just so you don't completely blow their minds.
Mental squares23/2/2015 Thank you, Internet, for wasting my evening. I don't know how I found this on YouTube - one of my random click-a-thons searching for goodies, and I'm a sucker for "magic maths" tricks. This one is how to square any (two-digit) number mentally. If you don't want to watch the video, here's the basic idea with 33² as an example:
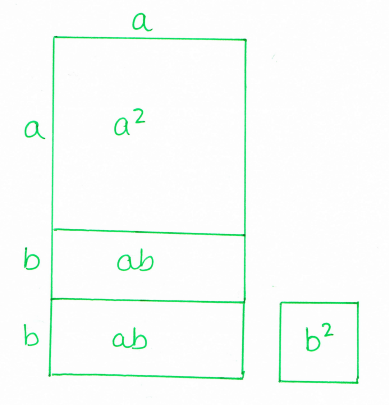
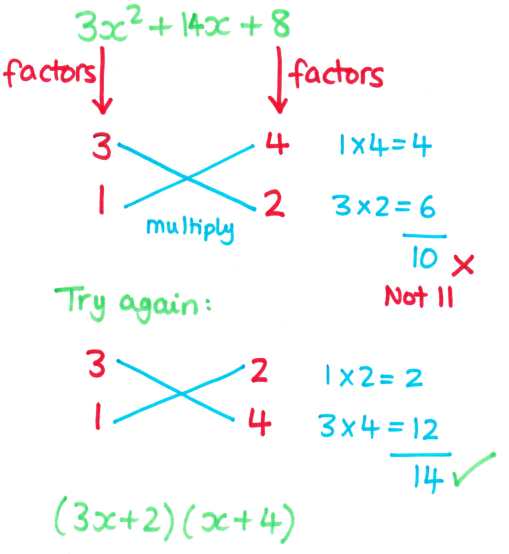
And here's my workings: So I did this one...then tried another. Cute? Convoluted, really, and not particularly workable in the curriculum (I'd much prefer a sensible approach with proper multiplication), but then I got one of those "oh now how does that work?" moments. I could see how it was kinda linked to quadratics and square areas, so I did some doodling. After many aborted attempts, I came up with this: Take a square with side length 33(cm if you insist on units). You can then chop a rectangle off the edge of length 3 (making an easier multiplication of 30 x something) and whack that on the bottom. So then you've got a 30 x 36 rectangle, and the little square left over to add on. Then, because I'm a mathematician and I like doing things properly, I tried to make it work with algebra: Typical quadratic expansion, but we think about a as the tens digit and b as the units, because we're trying to change the square into a rectangle with length a (so we have a multiple of 10 to calculate with). Then: So we've changed the problem from (a + b)² (difficult) to a(a + 2b) + b² (messy but easier mentally). I was quite pleased with this! Just thought this might be a nice challenge problem to give to pupils when working with quadratics (or square numbers for that matter) - show them the video, then get them to explain why it works - numerical or algebraic approach. I do think this lends itself to an exploration of the link between square numbers, area and quadratics, which I think is often overlooked. Factorising quadratics is one of those topics that, for some reason, students just don't seem to get. I always teach factorising straight after expanding; it seems that students understand the basic principle of "working backwards", and can even factorise fairly simple quadratics (all positives, of course) pretty quickly and simply. However, I've never quite managed to work my way around more difficult quadratics with a > 1 with much success; the students seem to understand what they are trying to do, but really struggle with putting it into practice. Anyway, last week I went on a training course for the new 9-1 GCSE from Edexcel. All interesting stuff about the exam changes, but my biggest takeaway was this: the "Cross Method" for factorising quadratics with a > 1. I was keen to give it a go with Year 10, so after doing some simple quadratics with a = 1 (mostly OK, apart from the usual wrangles with negative numbers and having to unteach negative add negative is positive), we tried a few with a > 1. I started with my usual "reverse grid method and reason it out" approach; blank stares and confusion. I then showed them the same question using the "Cross Method". Cue shouts of "oh that's easy!" and "why did you even show us that first way?!". Things I like about this method:
I think I'll be using this a lot more in future! It's not a substitute for understanding, but once the understanding is in place, it provides structure and organisation for the trial and error method. |
Categories
All
Archives
August 2020
|
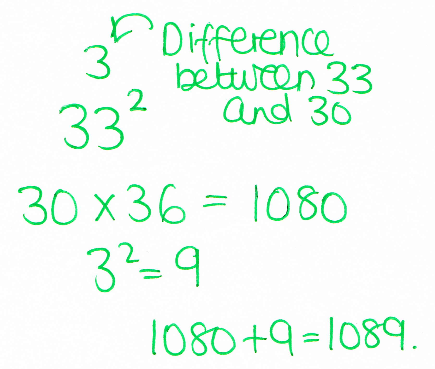
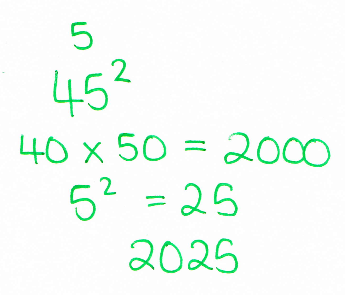

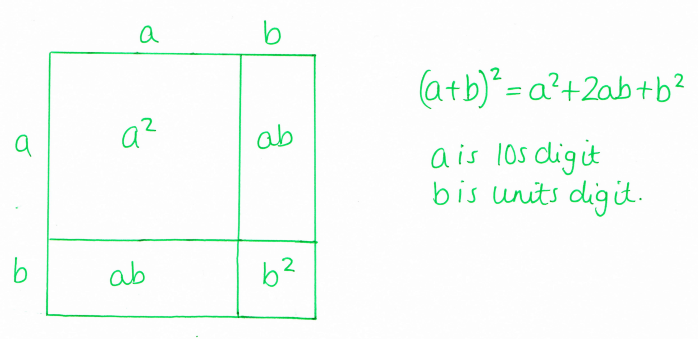
 RSS Feed
RSS Feed
