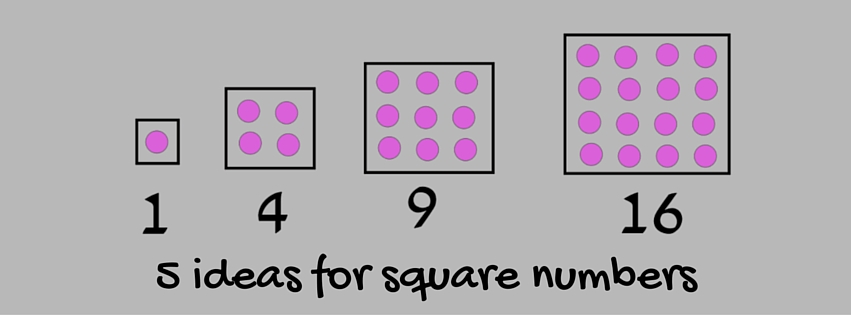
These are all great investigations/rich problems with plenty of practice working with square numbers - a little more interesting than fifteen questions out of a textbook. If pupils have to learn facts, such as the squares to 15, I think it's better to embed them into something a little mathematically rich than just drill.
Five ideas for square numbers25/2/2016 Post 25/29 in the Staffrm #29daysofwriting challenge: Lesson ideas for exploring square numbers As we've got two squares in today's date, I thought I'd go for a square themed post. Also I've just realised that this year contains 25/04/16, and 25/09/16, both even better excuses for a lesson on square numbers.
These are all great investigations/rich problems with plenty of practice working with square numbers - a little more interesting than fifteen questions out of a textbook. If pupils have to learn facts, such as the squares to 15, I think it's better to embed them into something a little mathematically rich than just drill. I thought Christmas was over...?4/2/2016 Mental squares23/2/2015 Thank you, Internet, for wasting my evening. I don't know how I found this on YouTube - one of my random click-a-thons searching for goodies, and I'm a sucker for "magic maths" tricks. This one is how to square any (two-digit) number mentally. If you don't want to watch the video, here's the basic idea with 33² as an example:
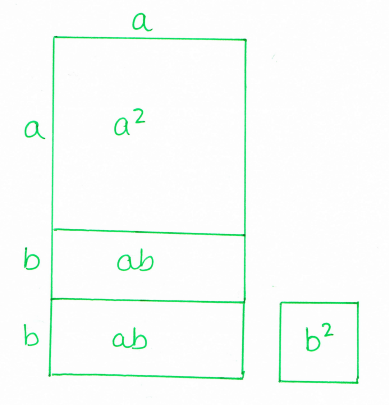
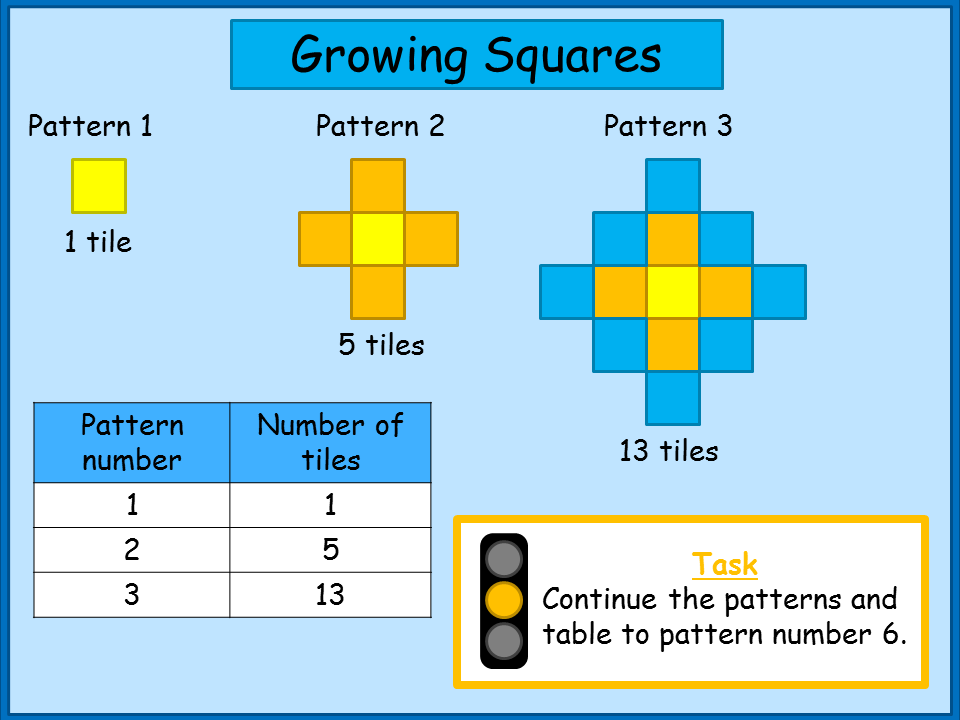
And here's my workings: So I did this one...then tried another. Cute? Convoluted, really, and not particularly workable in the curriculum (I'd much prefer a sensible approach with proper multiplication), but then I got one of those "oh now how does that work?" moments. I could see how it was kinda linked to quadratics and square areas, so I did some doodling. After many aborted attempts, I came up with this: Take a square with side length 33(cm if you insist on units). You can then chop a rectangle off the edge of length 3 (making an easier multiplication of 30 x something) and whack that on the bottom. So then you've got a 30 x 36 rectangle, and the little square left over to add on. Then, because I'm a mathematician and I like doing things properly, I tried to make it work with algebra: Typical quadratic expansion, but we think about a as the tens digit and b as the units, because we're trying to change the square into a rectangle with length a (so we have a multiple of 10 to calculate with). Then: So we've changed the problem from (a + b)² (difficult) to a(a + 2b) + b² (messy but easier mentally). I was quite pleased with this! Just thought this might be a nice challenge problem to give to pupils when working with quadratics (or square numbers for that matter) - show them the video, then get them to explain why it works - numerical or algebraic approach. I do think this lends itself to an exploration of the link between square numbers, area and quadratics, which I think is often overlooked. Growing squares29/1/2015
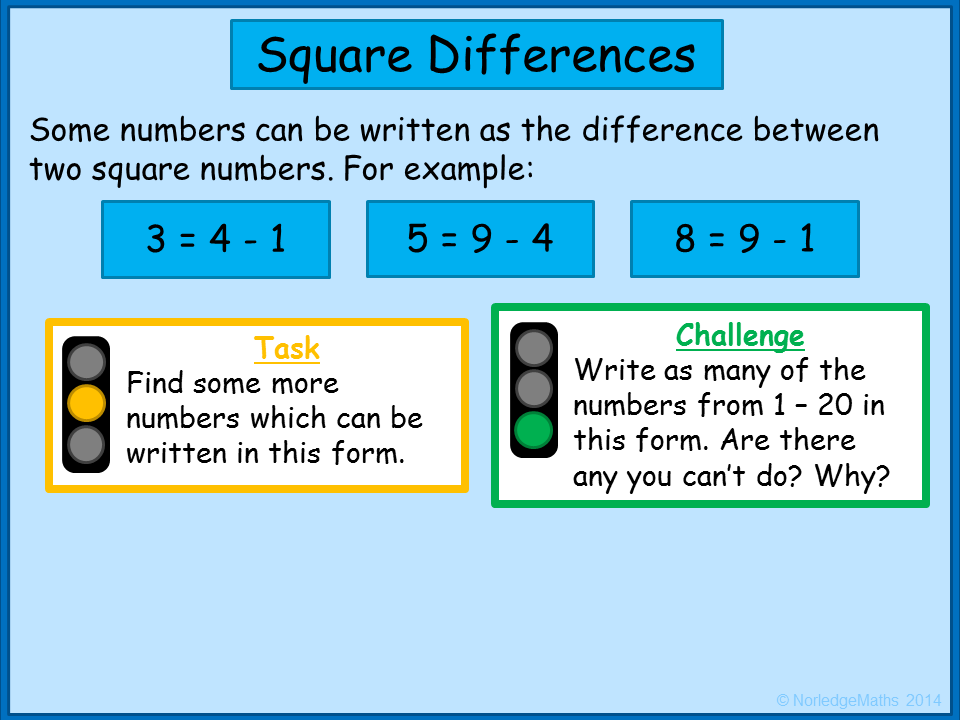
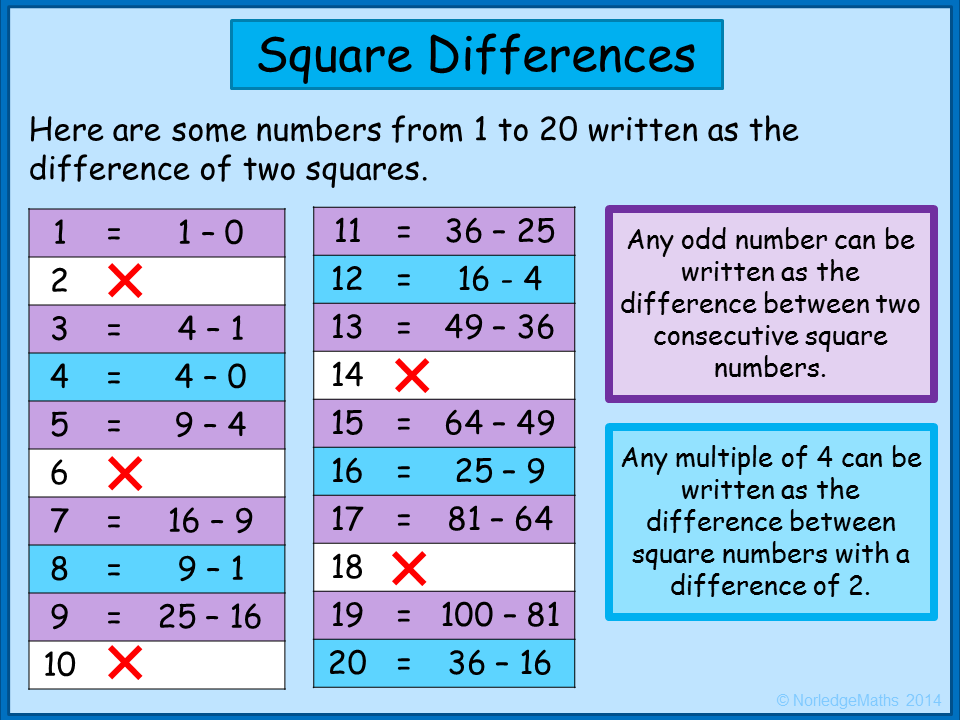
Square differences28/1/2015
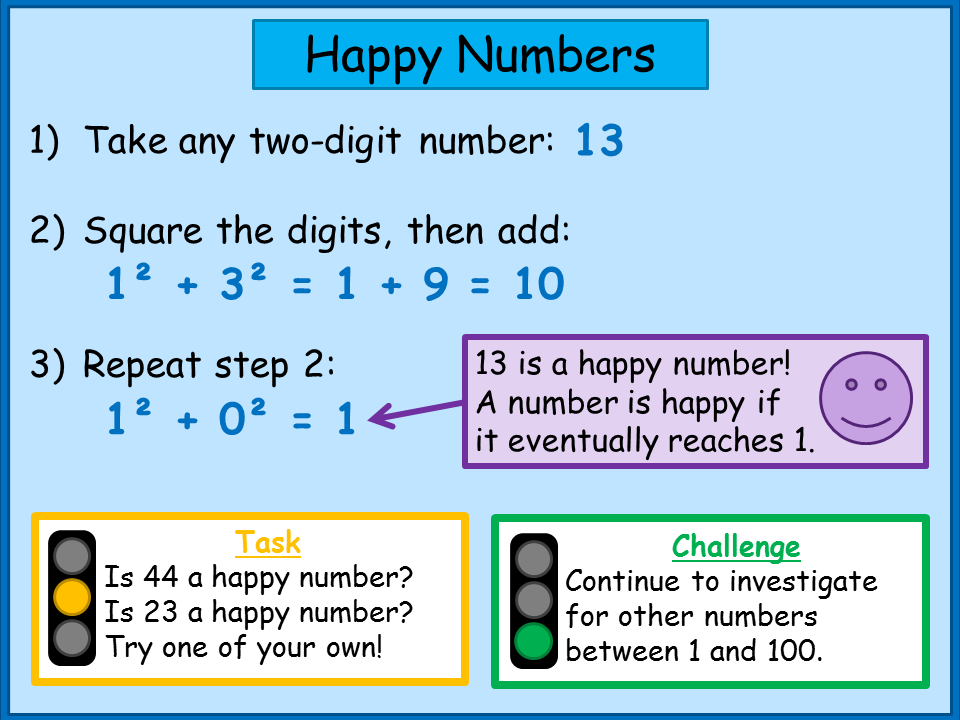
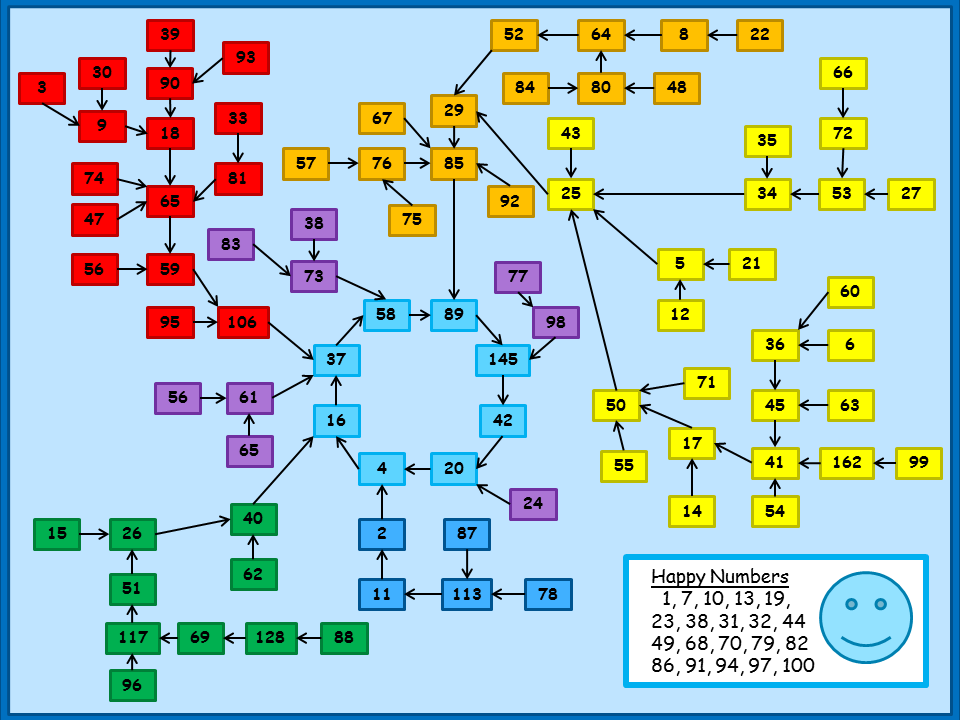
Happy numbers28/1/2015
|
Categories
All
Archives
August 2020
|


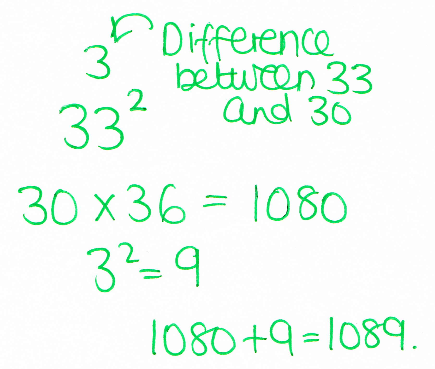
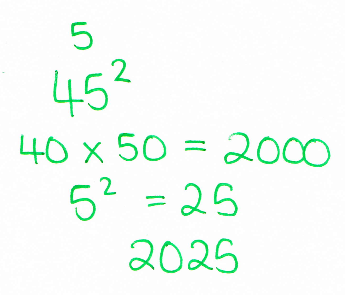

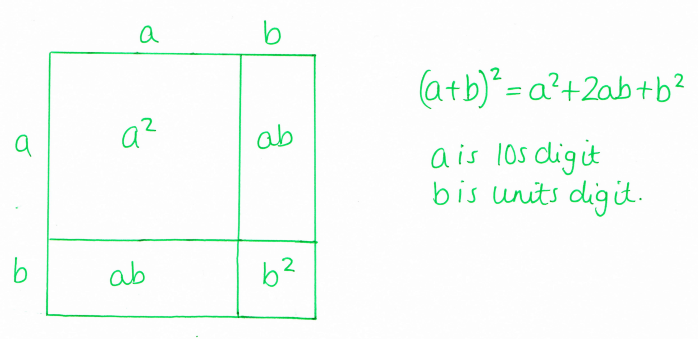
 RSS Feed
RSS Feed
