Love is in the air!14/2/2016 Post 14/29 in the Staffrm #29daysofwriting challenge: Sentimentality and graph of a heart When I was younger, I was ridiculously cynical about Valentine's Day - I'd wonder why people needed one special day to tell or show their significant other how much they love them, when surely that should be happening all the time. However, as I'm often working late into the evenings (I get in at 4pm but usually do 2-3 hours at home in the evening), sometimes I find my husband's come in at 5 and I've not even acknowledged him, because I'm so engrossed in school-work. It's very easy to neglect to remind the people who are important to you just how important they are - family and friends as well as significant others.
Making money5/2/2016 Six good comics and one good thing27/8/2015 It's only a model - Part 22/8/2015 Another quick one today to follow on from yesterday's post about the best maths in action I've ever seen, with the promise that more relevant blogging will resume from tomorrow! Here are a couple of pics of the "scale model" in action... It's only a model...1/8/2015
How does Bob Marley like his Maths...?22/7/2015 ...With jam in! Badumdum-tshh...
Not sure if that really works, but I enjoyed my first Maths Jam so much last night that I thought it would make a great topic for my SBPC post today. If you don't know about Maths Jam (I didn't until Beth (@MissBLilley) invited me along at the last maths conference), it's a monthly get-together for "maths enthusiasts" in your local area, which understandably attracts a lot of maths teachers. It's also something I wish I'd found out about a lot sooner, as I've been back in Leeds for over four years now, and would have really enjoyed going along to these as I was settling back in and making new friends. Warning: This post contains spoilers for a couple of problems from Solve my Maths. Bit of a random topic for my third SBPC, but bear with me. Over the weekend, my fiance dragged me to watch Jurassic World; I wasn't keen, as I missed the Jurassic Park hype when I was younger, and don't really enjoy action films. Since watching Jurassic World, we went back and watched three episodes of Planet Dinosaur - I'd never seen it before and found it fascinating, although the CGI looks a little dated now!
While we were watching it, we ended up discussing the huge time frames involved in the evolution of both dinosaurs and life in general. When I visualise dinosaurs, I imagine all the famous ones like Tyrannosaurus Rex, Stegasaurus and Diplodocus all roaming the planet at the same time. Something that struck me was the phenomenal length of time that dinosaurs in general were on the planet, and the (relative) shortness of the time that each recognisable species was around.
Following my trawl through the xkcd archives to find my favourite comics for A Level, I thought I may as well post some that may be relevant for KS3 and GCSE. There are plenty of funny graphs and pie charts (great for the last five minutes of a lesson), and a few topic-related strips.
This post contains a few percentages comics; while they're quite funny, they also have quite a bit of mileage in terms of mathematical discussion, particularly "Hand Sanitizer" and "Fastest Growing". Clicking on each picture will take you to the xkcd site, where you can get larger resolution versions. xkcd strips for A Level22/3/2015 If you haven't heard of xkcd (click now and lose three hours), it's a webcomic run by Randall Monroe focusing on maths, science and computing. Although some of his comics (mostly the coding ones) go over my head, the maths-based ones are hilarious, and some are great for sharing in lessons - with the caveat that some do contain naughty jokes, so check first. Here's my pick of a few particularly relevant to the A Level syllabus:
Through the Looking Glass (Part 1)25/2/2015 It arrived! Having ordered a copy of Alex Bellos's second book, Alex Through the Looking Glass right after discovering it existed on 11th February, the gods at Amazon finally decided to deposit it on my doormat this afternoon. I nearly crushed it when I opened the door... So after hacking into it with an over-large knife (couldn't find the scissors) and discarding the packaging in a very haphazard fashion, I started reading. I've read three chapters tonight, on and off, and am going to finish Chapter 4 before I go to bed (although it does appear to be about conic sections...urrrrgh). I absolutely loved his first book (Adventures in Numberland) and have read it cover to cover at least three or four times, and dipped into it for lesson ideas (it's looking very dog-eared now) and I'm pleased to say I'm enjoying this one just as much. So far this evening I've discovered:
If you like maths books, particularly those which don't give you a headache because the maths requires you to dig out university notes to understand the first paragraph, I'm recommending this. Of course, I'm only three chapters in, and the bit on conic sections may kill me (3D geometry is not my favourite thing ever). Wish me luck! Mental squares23/2/2015 Thank you, Internet, for wasting my evening. I don't know how I found this on YouTube - one of my random click-a-thons searching for goodies, and I'm a sucker for "magic maths" tricks. This one is how to square any (two-digit) number mentally. If you don't want to watch the video, here's the basic idea with 33² as an example:
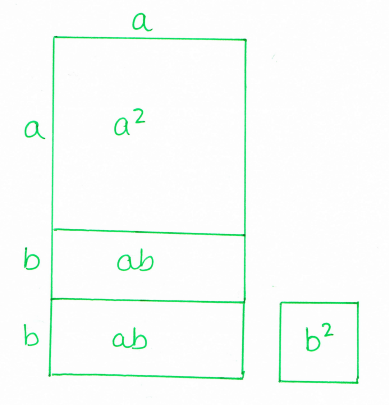
And here's my workings: So I did this one...then tried another. Cute? Convoluted, really, and not particularly workable in the curriculum (I'd much prefer a sensible approach with proper multiplication), but then I got one of those "oh now how does that work?" moments. I could see how it was kinda linked to quadratics and square areas, so I did some doodling. After many aborted attempts, I came up with this: Take a square with side length 33(cm if you insist on units). You can then chop a rectangle off the edge of length 3 (making an easier multiplication of 30 x something) and whack that on the bottom. So then you've got a 30 x 36 rectangle, and the little square left over to add on. Then, because I'm a mathematician and I like doing things properly, I tried to make it work with algebra: Typical quadratic expansion, but we think about a as the tens digit and b as the units, because we're trying to change the square into a rectangle with length a (so we have a multiple of 10 to calculate with). Then: So we've changed the problem from (a + b)² (difficult) to a(a + 2b) + b² (messy but easier mentally). I was quite pleased with this! Just thought this might be a nice challenge problem to give to pupils when working with quadratics (or square numbers for that matter) - show them the video, then get them to explain why it works - numerical or algebraic approach. I do think this lends itself to an exploration of the link between square numbers, area and quadratics, which I think is often overlooked. The Lottery, lemmings and primes (COM15)11/2/2015 I have to admit, I was flagging by this point in the afternoon. A combination of an earlier-than-usual start on a Saturday and an inevitable caffeine crash and accompanying headache at about half three meant I was actually (shamefully) considering disappearing early - but I am SO glad I stuck around for Marcus du Sautoy's lecture to finish the day. I'm a big fan of School of Hard Sums, and I love some of the TV shows that Marcus has done, so I knew this would be good. What surprised me was just how exciting it was being in a room full of enthusiastic mathematicians listening to a lecture from an enthusiastic mathematician; it's this joy that I wish we could bottle and give to students somehow. The hour flew by, and I left with a spring in my step and a reminder of why I love maths so much. Marcus started the talk by showing us some sequences, and talking about how mathematics was about pattern-spotting. I got the first couple of sequences (I love how I turn into a school child again whenever anyone asks me a maths question and I get excited because I know the answer), but the sequence 1, 2, 4, 8, 16, ... threw me and everyone else in the audience. You'd assume the answer was 32, but it's actually 31, because this is the sequence of circle division numbers. Quite a nice illustration of how mathematical patterns can be deceptive! Another interesting point from this is the use of the Fibonacci (why can I NEVER spell that) in music, or more accurately, the fact that the so-called "Fibonacci" sequence was being used for beat counts in Indian music way before Leonardo gave his name to it. @Kirstymaths tweeted a pic of this that's worth checking out. Marcus then talked about prime numbers, and suggested a more intuitive reason that 1 isn't a prime number. If we think of the primes as building blocks for all the other integers (Fundemental Theorem of Arithmetic), then 1 isn't prime because we can't make anything with it. It's interesting that mathematicians have flipped back and forth on 1 for years; I vaguely remember something from my Numbers and Algebra course in the third year of my degree, but Wikipedia is much more accessible than the lecture notes gathering dust in my loft. The lecture then progressed to talking about cicadas, insects whose life-cycle lasts a prime number of years. I remember finding out about this last year when a friend in America sent me a video very similar to this one of cicadas in his local area; I was amazed at the volume the insects create, and even more amazed when I read some of the linked news articles that explained that these particular cicadas emerge once every 17 years. There are some theories that this is to do with the cycles of now-extinct predators; choosing a prime numbered life cycle would mean that the cicada has less chance of meeting a surge of predators whose life cycle works on multiples of 2 or 4 (for example). I've also just found a nice video from the BBC's Life in the Undergrowth narrated by David Attenborough - I'm feeling inspiration for a lesson on primes and LCM here! We then did a lottery activity, and Marcus predicted (pretty accurately) how many of us would have 1, 2 3 or 4 numbers correct. He talked about the ideas that people don't select consecutive numbers because they think these are less likely, but pointed out that half of all possible choices contain consecutive numbers. He also talked about the 1, 2, 3, 4, 5, 6 selection - I love using this when I do combinations with Year 12, and having a discussion about how you think you're clever because (if you're a mathematician) you know that this is equally as likely as any other possible combination, but how you'd be kicking yourself if you won, as there are (apparently) about ten thousand people in the country who pick this per week, and you'd be splitting the prize more than if you went for 22, 23, 24, 25, 26, 27. Marcus finished with a lovely demonstration of patterns in populations, looking at one model for lemmings to explain the four-year "suicide". Thanks to QI (about 20 minutes in, profanity warning), I was already pretty clued up on the lemming myth (another proud schoolkid moment!), but Marcus demonstrated (using quite a simple mathematical model) how a population could stabilise, then changed this model to show how the lemming population could vary wildly to explain the four-year dip. Again, tempted to try this in the classroom! I'm sure there's lots of stuff I've missed, but this was a brilliant way to end the day. Like I said at the start, there's something infectious about being in a room full of people who love maths and are enjoying themselves doing or thinking about mathematics, and it's a shame that this doesn't translate to our students sometimes.
|
Categories
All
Archives
August 2020
|













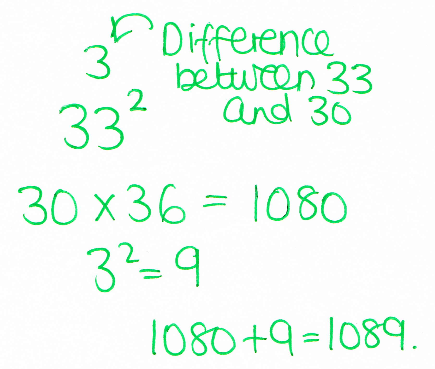
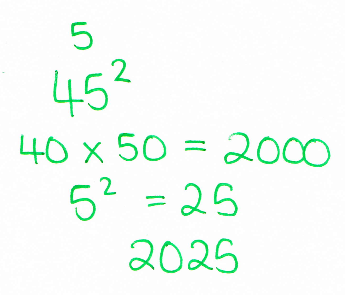

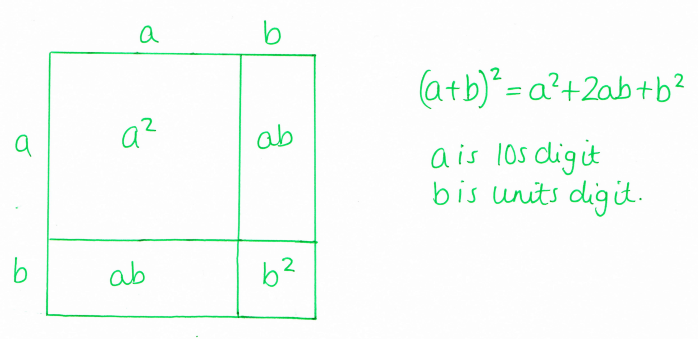

 RSS Feed
RSS Feed
