Two major changes this year have been use of the bar model (read about my discovery here) and linking cubes. I'm trying to do a lot more in concrete situations before moving to rules, and using manipulatives and models seems to get the ideas to stick.
1. Start with a chocolate bar
Think Cadbury's Dairy Milk (single bar), Kinder Bueno, Hershey Bars - anything which comes in a single row of squares or pieces of chocolate. The first thing I did was show the students some pictures of these chocolate bars, and get them to tell me each piece size as a fraction - e.g. the Bueno has four pieces, so if I eat one piece, I've eaten 1/4, two pieces is 2/4 and so on. We spent some time discussing what the numerator and denominator tell us about the different chocolate bars, and emphasising that the denominator told us about the size of one whole bar.
2. Move to a physical model
I then gave students different amounts of link cubes, explaining that they were pretending these were squares of chocolate. I asked them to make me as many whole chocolate bars as they could - we used the Bueno 4 square model to start with. There were a few comments about how I'd not given them enough cubes in some cases (they were expecting multiples of four); I kept quiet and just told them to build what they could. Once they'd built for a bit, a couple of the students realised that this was the point of the task.
We then collected ideas on the board and looked at different students' examples. I deliberately started using a bar model at this point to represent the cube pictures they were explaining. This is also a good point to get students to draw their models on the board themselves and explain what they've done.
3. Introduce improper fraction notation
I took one example and modeled the use of fraction notation, drawing on our original chocolate examples. So 13/4 means 13 pieces of chocolate, and one whole bar has 4 pieces. We then discussed how this related to mixed number form:
- How many whole chocolate bars? This is the big number.
- How many pieces that don't make a whole bar? This is the numerator of the fraction part.
- How many pieces in a whole bar? This is the denominator.
4. Practise problems using cubes and diagrams
I then gave students some problems to try for themselves. They started with more examples using four-square chocolate bars, then progressed to other sizes. I kept denominators fairly small to start with (2, 3, 4 and 5), just because modelling and drawing gets a little impractical with larger denominators. I encouraged students to use the cubes where necessary, and insisted they drew diagrams for the first few they attempted.
5. Work the opposite way
Some of the classes I've tried this with were ready to move on to converting back the other way, either with diagrams or by applying understanding gained from working from improper to mixed within the same lesson.
Some of them needed a little more consolidation work one way before we moved on. One lesson I'm learning this year is how important it is not to push too quickly; a new idea takes time to cement thoroughly, and I think it's worth working on one thing properly at a time, rather than charging ahead and progressively losing students along the way.
If you're looking for questions quickly, Math Aids has a great worksheet generator, differentiable by easy, medium and hard denominators.
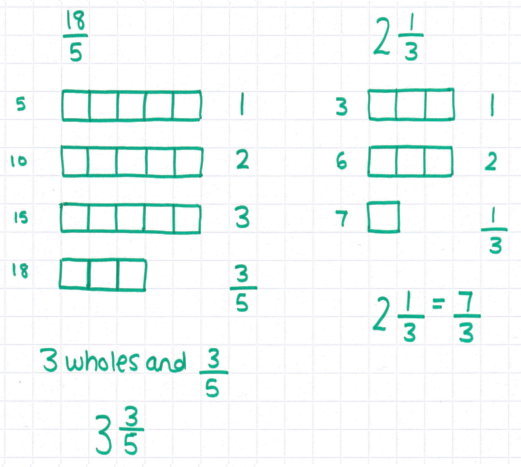
 RSS Feed
RSS Feed

When I was in elementary school, I was taught to multiply the denominator to the whole number and then add the numerator. The resulting number would be the new numerator now. That is the way to convert mixed number to improper fraction. This rule has has been implanted in my mind even though I am 37 now.
Converting mixed numbers to improper fractions is the first step before multiplying them.